已知 \(F_1\),\(F_2\) 是椭圆和双曲线的公共焦点,\(P\) 是它们的一个公共点,且 \(\angle F_1PF_2=\dfrac{\pi}{3}\),则椭圆和双曲线的离心率的倒数之和的最大值为(\(\qquad\))
A.\(\dfrac{4\sqrt3}{3}\)
B.\(\dfrac{2\sqrt3}{3}\)
C.\(3\)
D.\(2\)
解 法一 设椭圆的基本量为 \(a_1\),\(c\),离心率为 \(e_1\);双曲线的基本量为 \(a_2\),\(c\),离心率为 \(e_2\),则题中所求即为 \[\dfrac{1}{e_1}+\dfrac{1}{e_2}=\dfrac{a_1+a_2}{c}.\cdots\cdots①\]不妨设点 \(P\) 为椭圆与双曲线在第一象限的公共点,如图所示.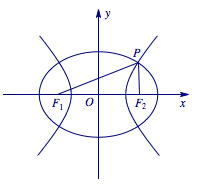 由椭圆与双曲线的定义,可得 \[\begin{cases}|PF_1|+|PF_2|=2a_1,\\|PF_1|-|PF_2|=2a_2,\end{cases}\] 两式相加,得\[a_1+a_2=|PF_1|.\cdots\cdots②\] 将②式代入①式,得 \[\dfrac{1}{e_1}+\dfrac{1}{e_2}=\dfrac{|PF_1|}{c}=2\cdot \dfrac{|PF_1|}{|F_1F_2|}.\] 在 \(\triangle PF_1F_2\) 中,我们运用正弦定理,得 \[\dfrac{|PF_1|}{|F_1F_2|}=\dfrac{\sin\angle PF_2F_1}{\sin\angle F_1PF_2}=\dfrac{2}{\sqrt3}\cdot \sin\angle PF_2F_1.\] 由此,不难得出,当 \(\angle PF_2F_1=\dfrac{\mathrm\pi}{2}\) ,可解得 \(e_1=\dfrac{\sqrt3}{3}\),\(e_2=\sqrt3\) 时,题目所求取得最大值为 \(\dfrac{4\sqrt3}{3}\),选项 A 正确.
由椭圆与双曲线的定义,可得 \[\begin{cases}|PF_1|+|PF_2|=2a_1,\\|PF_1|-|PF_2|=2a_2,\end{cases}\] 两式相加,得\[a_1+a_2=|PF_1|.\cdots\cdots②\] 将②式代入①式,得 \[\dfrac{1}{e_1}+\dfrac{1}{e_2}=\dfrac{|PF_1|}{c}=2\cdot \dfrac{|PF_1|}{|F_1F_2|}.\] 在 \(\triangle PF_1F_2\) 中,我们运用正弦定理,得 \[\dfrac{|PF_1|}{|F_1F_2|}=\dfrac{\sin\angle PF_2F_1}{\sin\angle F_1PF_2}=\dfrac{2}{\sqrt3}\cdot \sin\angle PF_2F_1.\] 由此,不难得出,当 \(\angle PF_2F_1=\dfrac{\mathrm\pi}{2}\) ,可解得 \(e_1=\dfrac{\sqrt3}{3}\),\(e_2=\sqrt3\) 时,题目所求取得最大值为 \(\dfrac{4\sqrt3}{3}\),选项 A 正确.
法二 不妨假设椭圆与双曲线的公共焦点 \(c=1\),\(|PF_1|=m\),\(|PF_2|=n\),则由法一可知,\[\dfrac{1}{e_1}+\dfrac{1}{e_2}=a_1+a_2=m.\] 在三角形 \(PF_1F_2\) 中,有余弦定理 \[|F_1F_2|^2=|PF_1|^2+|PF_2|^2-2|PF_1||PF_2|\cos\angle F_1PF_2,\] 即 \[4=m^2+n^2-mn,\] 将上式中 \(n\) 看成主元,\(m\) 看成参数,整理为二次方程 \[n^2-mn+(m^2-4)=0,\] 因此,上述方程有解,需判别式大于等于零,即 \[\Delta=m^2-4(m^2-4)\geqslant0,\] 解得 \(m\leqslant\dfrac{4\sqrt3}{3}\),当 \(m=\dfrac{4\sqrt3}{3}\) 时, \(n=\dfrac{2\sqrt3}{3}\),\(e_1=\dfrac{\sqrt3}{3}\),\(e_2=\sqrt3\),选项 A 正确.
