已知矩形ABCD中AB=√2BC,以CD为直径向矩形外作半圆,设P为半圆上任意一点,直线PA,PB分别与CD相交于点M,N,求证:CD2=CM2+DN2.
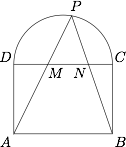
 证明 以CD的中点为原点,DC为x轴方向建立平面直角坐标系,不妨设AB=2,如图.
证明 以CD的中点为原点,DC为x轴方向建立平面直角坐标系,不妨设AB=2,如图.
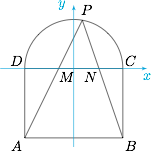 设P(cosθ,sinθ),A(−1,−√2),B(1,−√2),C(1,0),D(−1,0),于是根据截距坐标公式,M,N的横坐标分别为xM=cosθ⋅(−√2)−(−1)⋅sinθ−√2−sinθ=−sinθ+√2cosθ√2+sinθ,xN=cosθ⋅(−√2)−1⋅sinθ−√2−sinθ=sinθ+√2cosθ√2+sinθ,于是CM2+DN2=(1−xM)2+(xN−(−1))2=(√2+2sinθ−√2cosθ)2+(√2+2sinθ+√2cosθ)2(√2+sinθ)2=4=CD2,因此原命题得证.
设P(cosθ,sinθ),A(−1,−√2),B(1,−√2),C(1,0),D(−1,0),于是根据截距坐标公式,M,N的横坐标分别为xM=cosθ⋅(−√2)−(−1)⋅sinθ−√2−sinθ=−sinθ+√2cosθ√2+sinθ,xN=cosθ⋅(−√2)−1⋅sinθ−√2−sinθ=sinθ+√2cosθ√2+sinθ,于是CM2+DN2=(1−xM)2+(xN−(−1))2=(√2+2sinθ−√2cosθ)2+(√2+2sinθ+√2cosθ)2(√2+sinθ)2=4=CD2,因此原命题得证.

第一行式子肯定打错了。显然应该是AB=sqr(2)*BC吧。
已改,谢谢