(理8)已知函数f(x)={x2−x+3,x⩽1,x+2x,x>1,设a∈R，若关于x的不等式f(x)⩾|x2+a|在R上恒成立，则a的取值范围是( )
A．[−4716,2]
B．[−4716,3916]
C．[−2√3,2]
D．[−2√3,3916]
分析与解 A．
根据题意，有∀x∈R,−f(x)−x2⩽a⩽f(x)−x2,因此只需要计算函数g(x)=−f(x)−x2在R上的最大值和函数h(x)=f(x)−x2在R上的最小值即可．函数g(x)即g(x)={−x2+x2−3,x⩽1,−32x−2x,x>1,其最大值为max函数h(x)即h(x)=\begin{cases} x^2-\dfrac 32x+3,&x\leqslant 1,\\ \dfrac x2+\dfrac 2x,& x>1,\end{cases}其最小值为\min\{h(x)\}=\min\left\{\dfrac{39}{16},2\right\}=2.因此所求的取值范围是\left[-\dfrac{47}{16},2\right]．
(理14)用数字1,2,3,4,5,6,7,8,9组成没有重复数字，且至多有一个数字是偶数的四位数，这样的四位数一共有______个．(用数字作答)
分析与解 1080．
情形一 四位数中没有偶数数字，个数为{\rm A}_5^4=120.
情形二 四位数中有一个偶数数字，个数为{\rm C}_4^1{\rm C}_5^3{\rm A}_4^4=960.
因此符合题意的四位数共有120+960=1080个．
(理19)设椭圆\dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1(a>b>0)的左焦点为F，右顶点为A，离心率为\dfrac 12．已知A是抛物线y^2=2px(p>0)的焦点，F到抛物线的准线l的距离为\dfrac 12．
(1)求椭圆的方程和抛物线的方程；
(2) 设l上两点P,Q关于x轴对称，直线AP与椭圆相交于点B(B异于点A)，直线BQ与x轴相交于点D，若\triangle APD的面积为\dfrac{\sqrt 6}2，求直线AP的方程．
分析与解 (1) 根据题意，抛物线的准线l过椭圆的左顶点，因此有\begin{cases}\dfrac ca=\dfrac 12,\\ a-c=\dfrac 12,\end{cases}其中c为椭圆的半焦距．解方程，可得椭圆的方程为x^2+\dfrac{4y^2}3=1,抛物线的方程为y^2=4x.
(2) 如图．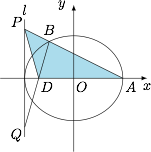
考虑到图形的对称性，先计算B点纵坐标为正实数的情形．设B\left(\cos\theta,\dfrac{\sqrt 3}2\sin \theta\right)，P(-1,m)，则\dfrac{m-0}{-1-1}=\dfrac{\dfrac{\sqrt 3}2\sin\theta-0}{\cos\theta-1},解得m=\dfrac{\sqrt{3}\sin\theta}{1-\cos\theta}.于是Q\left(-1,\dfrac{\sqrt 3\sin\theta}{\cos\theta-1}\right)，进而可得D点的横坐标为\dfrac{\cos\theta\cdot \dfrac{\sqrt 3\sin\theta}{\cos\theta-1}-(-1)\cdot\dfrac{\sqrt 3}2\sin\theta}{\dfrac{\sqrt 3\sin\theta}{\cos\theta-1}-\dfrac{\sqrt 3}2\sin\theta}=\dfrac{3\cos\theta-1}{3-\cos\theta}.这样得到\triangle APD的面积\begin{split} S=&\dfrac 12\cdot \left(1-\dfrac{3\cos\theta-1}{3-\cos\theta}\right)\cdot \dfrac{\sqrt 3\sin\theta}{1-\cos\theta}\\=&\dfrac{2\sqrt3\sin\theta}{3-\cos\theta}=\dfrac{\sqrt 6}2,\end{split} 解得\cos\theta=\dfrac 13，\sin\theta=\dfrac{2\sqrt 2}3．进而可得直线AP的方程为y=-\dfrac{\sqrt 6}2x+\dfrac{\sqrt 6}2.
结合图形的对称性，可得直线AP的方程为y=-\dfrac{\sqrt 6}2x+\dfrac{\sqrt 6}2或y=\dfrac{\sqrt 6}2x-\dfrac{\sqrt 6}2．
(理20)设a\in\mathbb Z，已知定义在\mathbb R上的函数f(x)=2x^4+3x^3-3x^2-6x+a在区间(1,2)内有一个零点x_0，g(x)为函数f(x)的导函数．
(1) 求g(x)的单调区间；
(2) 设m\in [1,x_0)\cup (x_0,2]，函数h(x)=g(x)(m-x_0)-f(m)，求证：h(m)h(x_0)<0；
(3) 求证：存在大于0的常数A，使得对于任意的正整数p,q，且\dfrac pq\in [1,x_0)\cup (x_0,2]，满足\left|\dfrac pq-x_0\right|\geqslant \dfrac{1}{Aq^4}．
分析与解 (1) 函数g(x)为g(x)=8x^3+9x^2-6x-6,其导函数为g'(x)=24x^2+18x-6=6(4x-1)(x+1),因此函数g(x)的单调递增区间为(-\infty,-1)和\left(\dfrac 14,+\infty\right)；单调递减区间为\left(-1,\dfrac 14\right)．
(2) 令\varphi(x)=g(x)(x-x_0)-f(x)，则其导函数\varphi'(x)=g'(x)(x-x_0),而在[1,2]上，g'(x)>0，因此\varphi(x)在[1,x_0)上单调递减，在(x_0,2]上单调递增，进而在[1,x_0)\cup (x_0,2]上，\varphi(x)>0，故h(m)=\varphi(m)>0.
令\mu(x)=g(x_0)(x-x_0)-f(x)，则其导函数\mu'(x)=g(x_0)-g(x),而在[1,2]上，g(x)单调递增，因此\mu(x)在[1,x_0)上单调递增，在(x_0,2]上单调递减，进而在[1,x_0)\cup (x_0,2]上，\mu(x)<0，故h(x_0)=\mu(m)<0.
综上所述，有h(m)\cdot h(x_0)<0，命题得证．
(3) 由于函数h(x)在[1,2]上单调，根据第(2)小题h(x)在m和x_0之间存在唯一零点．令m=\dfrac pq，h(x)在m和x_0之间的零点为x_1，则有h(x_1)=g(x_1)\left(\dfrac pq-x_0\right)-f\left(\dfrac pq\right)=0,进而\left|\dfrac pq-x_0\right|=\dfrac{\left|f\left(\dfrac pq\right)\right|}{g(x_1)}=\dfrac{\left|2p^4+3p^3q-3p^2q^2-6pq^3+aq^4\right|}{g(x_1)\cdot q^4},由于\dfrac pq\ne x_0，而函数f(x)在[1,2]内的零点唯一，因此\left|2p^4+3p^3q-3p^2q^2-6pq^3+aq^4\right|\ne 0,进而有\left|2p^4+3p^3q-3p^2q^2-6pq^3+aq^4\right|\geqslant 1,又因为g(x)在[1,2]上单调递增，所以g(x_1)\in[g(1),g(2)]=[5,82],因此取A=82即可，因此命题得证．