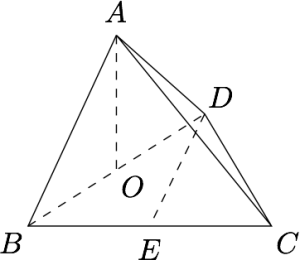
如图,四面体ABCD中,O,E分别是BD,BC的中点,AO垂直于平面BCD,且CA=CB=CD=2,AB=√2,求异面直线AB与ED所成角的大小.
分析与解 记→CA=2→a,→CB=2→b,→CD=2→c,
其中→a,→b,→c均为单位向量,则→CO=→b+→c,→CE=→b,→AO=→b+→c−2→a,
而AO垂直于平面BCD,于是(→b+→c−2→a)⋅→b=(→b+→c−2→a)⋅→c=0,
即→b⋅→c−2→a⋅→b+1=0,→b⋅→c−2→a⋅→c+1=0⋯⋯(i),
又AB=√2,于是(2→a−2→b)⋅(2→a−2→b)=2,即→a⋅→b=34⋯⋯(ii),
由(i)(ii),得→b⋅→c=12,→a⋅→c=34,
又 →AB=2→b−2→a,→ED=2→c−→b,于是异面直线AB与ED所成角θ满足cosθ=|(2→b−2→a)⋅(2→c−→b)|√2⋅√(2→c−→b)⋅(2→c−→b)=|4→b⋅→c−2−4→a⋅→c+2→a⋅→b|√2⋅√4−4→b⋅→c+1=√64,
因此,所求角为arccos√64.