已知函数$f(x)=a\ln x+\dfrac 1x+\dfrac 1{2x^2}$,$a\in\mathcal R$.
(1)讨论函数$f(x)$的单调性;
(2)证明:$(x-1)\left({\rm e} ^{-x}-x\right)+2\ln x<\dfrac 23$.
(1)解 $f(x)$的导函数$$f'(x)=\dfrac 1{x^3}\left(ax^2-x-1\right) ,$$进而可得:
当$a \leqslant 0$时,函数$f(x)$在$\mathcal R^+$上单调递减;
当$a>0$时,函数$f(x)$在$\left(0,\dfrac{1+\sqrt{1+4a}}{2a} \right) $上单调递减,在$\left(\dfrac{1+\sqrt {1+4a}}{2a},+ \infty \right) $上单调递增.
(2)证明 在(1)中取$a=2$,则可得$$2\ln x+\dfrac 1x+\dfrac 1{2x^2}\geqslant f(1)=\dfrac 32,$$将其中的$x$用$\dfrac 1x$替换,可得$$-2\ln x+x+\dfrac{x^2}2\geqslant \dfrac 32,$$即$$2\ln x\leqslant x+\dfrac{x^2}2-\dfrac 32.$$
将上述不等式代入欲证明不等式的左边,有$$\begin{split} (x-1)\left({\rm e} ^{-x}-x\right)+2\ln x &<(x-1)\cdot {\rm e} ^{-x}-x^2+x+\left(x+\dfrac{x^2}2-\dfrac 32\right)\\&=(x-1)\cdot {\rm e} ^{-x}-\dfrac{x^2}2+2x-\dfrac 32,\end{split} $$设右侧函数为$g(x)$,则$g(x)$的导函数$$g'(x)=(2-x)\left({\rm e} ^{-x}+1\right),$$于是$$g(x)\leqslant g(2)=\dfrac{1}{{\rm e} ^2} +\dfrac 12<\dfrac 23,$$因此原不等式得证.
事实上,如果不考虑利用(1)的结论.还可以尝试直接利用对数函数不等式$$\ln x\leqslant x-1$$进行放缩消去对数符号:$$\begin{split} (x-1)\left({\rm e} ^{-x}-x\right)+2\ln x&\leqslant (x-1)\left({\rm e} ^{-x}-x\right)+2(x-1)\\&=(x-1)\cdot {\rm e} ^{-x}+(2-x)(x-1).\end{split} $$
由于$$\left(\dfrac{x-1}{{\rm e} ^{x}}\right)'=\dfrac{2-x}{{\rm e} ^x},$$于是其最大值为$$\left. \left(\dfrac{x-1}{{\rm e} ^{x}}\right)\right|_{x=2}=\dfrac{1}{{\rm e} ^2},$$因此欲证明不等式左边$$ (x-1)\left({\rm e} ^{-x}-x\right)+2\ln x\leqslant \dfrac{1}{{\rm e} ^2}+\dfrac 14<\dfrac23.$$
注一 可以看到不用(1)的结论可以得到更好的结果.左侧函数的最大值约为$0.218797$,如图.
注二 湖南长沙周谊老师提供了一个更简单的作法:$$\begin{split} (x-1)\left({\rm e} ^{-x}-x\right)+2\ln x&\leqslant (x-1)\left({\rm e} ^{-x}-x\right)+2(x-1)\\&=(x-1)\cdot\left( {\rm e} ^{-x}-\dfrac{1}{\rm e} \right)+\left(2+\dfrac 1{\rm e} -x\right)(x-1)\\&<\dfrac 14\left(1+\dfrac{1}{\rm e} \right)^2&\\&<\dfrac 23.\end{split}$$


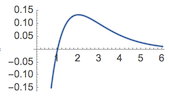
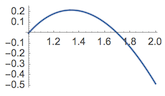
注一上方倒数第4行,在x=2处,(x-1)*e^-x取得最大值而不是最小值。
已更正,谢谢