如图,△ABC中,BA=BC,延长BA至点D使BD=AC,若∠BCD=50∘,求证:∠B=100∘.
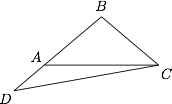
 分析与证明 设BD=AC=2,∠BDC=θ,则∠DCA=25∘−12θ,∠BCA=25∘+12θ.
分析与证明 设BD=AC=2,∠BDC=θ,则∠DCA=25∘−12θ,∠BCA=25∘+12θ.
在∠ADC中应用正弦定理,可得ACsin∠BDC=ADsin∠ACD=AC−ABsin∠ACD,
于是2sinθ=2−1cos(25∘+12θ)sin(25∘−12θ),
即2sin(25∘−12θ)⋅cos(25∘+12θ)=2cos(25∘+12θ)⋅sinθ−sinθ,
积化和差,得2sinθcos(25∘+12θ)=sin50∘.
设等式左边函数为f(θ),则其导函数f′(θ)=cosθ⋅cos(12θ+25∘)⋅[2−tanθ⋅tan(12θ+25∘)],
于是当θ在不超过50∘时,f(θ)单调递增,结合f(30∘)=sin50∘,可得θ=30∘,因此∠B=100∘.
注 本题来自尬题17,其中单调性的判断用到了tan50∘<√2的结论,下面给出这个结论的两种证明.
方法一 考虑到50∘的三倍150∘是个特殊角,考虑三倍角公式解决问题.
要证明tan50∘<√2即证明cos50∘>1√3,考虑到cos3x=4cos3x−3cosx,
知cos50∘是三次方程4x3−3x+√32=0
的根,当x=1√3时,方程左边小于零,所以cos50∘>1√3.
方法二 利用正切函数图象的性质,因为tan45∘=1,tan60∘=√3,所以tan50∘<13tan60∘+23tan45∘=2+√33<√2.
