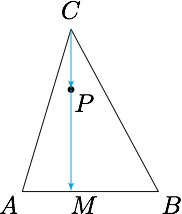
在$\triangle ABC$中，$A,B,C$所对的边分别为$a,b,c$，$M$为$AB$边上一点，$P$为直线$CM$上一点，且$\overrightarrow{CP}=\dfrac{\overrightarrow{CA}}{b\cos A}+\dfrac{\overrightarrow{CB}}{a\cos B}$，又已知$\left|\overrightarrow{CM}\right|=\dfrac c2$，$a^2+b^2=2\sqrt 2ab$，求$C$．
解 设$Q$是直线$CM$上不同于$C$点的任意一点，且$\overrightarrow{CQ}=x\overrightarrow{CA}+y\overrightarrow{CB}$，则$$\dfrac xy=\dfrac{BM}{AM},$$因此有$$\dfrac{BM}{AM}=\dfrac {a\cos B}{b\cos A}.$$
考虑到$a\cos B$与$b\cos A$的几何意义，作$AB$边上的高$CH$，则$$\dfrac{BH}{AH}=\dfrac{a\cos B}{b\cos A},$$于是点$M$即点$H$．这样有$\triangle ABC$的面积$$S=\dfrac 12 AB\cdot CH=\dfrac 14c^2,$$结合余弦定理，有$$\dfrac 14(a^2+b^2-2ab\cos C)=\dfrac 12ab\sin C,$$将$a^2+b^2=2\sqrt 2ab$代入，整理得$$\sin\left(C+\dfrac{\pi}4\right)=1,$$从而$C=\dfrac {\pi}4$．
注 也可以由$\overrightarrow {CP}\cdot\overrightarrow {AB}=0$得到$CM\perp AB$．